Dividing polynomials practice problems

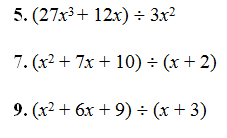
AB Divide by using long division. 14 575 56 15 14 1 41 First divide 14 into 57.

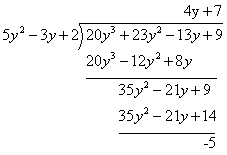
Use long division to divide 3x4 5x2 3 3 x 4 5 x 2 3 by x2 x 2.

Dividing polynomials practice problems. Section 5-1. For problems 1 3 use long division to perform the indicated division. Divide 3x4 - 5x2 3 by x 2 Solution.

Divide x3 2x2 - 3x 4 by x - 7 Solution. Divide 2x5 x4 - 6x 9 by x2 - 3x 1 Solution. Long division of Polynomials - Practice Problems.

Dividing by a Polynomial Containing More Than One Term Long Division Practice Problems. Move your mouse over the Answer to reveal the answer or click on the Complete Solution link to reveal all of the steps required for long division of polynomials. Now lets see with an example how to divide two polynomials Lets say we have px 2x 2 4x 1 and gx x 1.

Steps for long division. We will stop this process when the remainder becomes zero or its degree becomes less than divisor. Quotients first term is obtained by dividing the highest order term of dividend with the highest degree term of the divisor.

The lesson called Dividing Polynomials with Long and Synthetic Division. Practice Problems is a great resource you can use to learn more about this mathematical concept. In this lesson you will.

Synthetic Division of Polynomials - Practice Problems. Synthetic Division of Polynomials Practice Problems. Move your mouse over the Answer to reveal the answer or click on the Complete Solution link to reveal all of the steps required for synthetic division of polynomials.

Dividing Polynomials Practice and Problem Solving. AB Divide by using long division. 63xx x2 2.

2 10 5 5xxx x32 _____ _____ 3. 3 20 12 6 xx x2 4. 3 9 14 3xx x32 _____ _____ Divide by using synthetic division.

3 8 4 2xx x 2 6. 5 4 12 3xx x. First we will write this as a long division problem.

Now we will follow the steps above. DivideWe will divided the first term of the polynomial. So goes at the top as part of our answer.

We multiply the piece we just put as part of the answer by the entire binomial ð 2. Use long division to divide 3x4 5x2 3 3 x 4 5 x 2 3 by x2 x 2. Show All Steps Hide All Steps.

Lets first perform the long division. Just remember that we keep going until the remainder has degree that is strictly less that the degree of the polynomial were dividing by x 2 x 2 in this case. - Adding subtracting and multiplying polynomial expressions - Factoring polynomial expressions as the product of linear factors - Dividing polynomial expressions - Proving polynomials identities - Solving polynomial equations finding the zeros of polynomial functions - Graphing polynomial functions - Symmetry of functions.

To divide two polynomials we must apply a procedure called long division of polynomials. So lets see how to divide polynomials by polynomials using long division method by solving an example step by step. Calculate the result of dividing the following two polynomials.

The first thing we must do is place the polynomials in division form. To divide a polynomial by another polynomial use polynomial long division. This process is similar to long division using numbers.

Recall that to divide numbers you can use the following long division algorithm. 14 575 56 15 14 1 41 First divide 14 into 57. It goes in 4 times.

Multiply 4 by 14. Write the product 56 under 57. Subtract 56 from 57 to get 1.

Drop down the 5. Divide 14 into 15. It goes in 1 time.

Find first term by dividing the first term of the numerator by the first term of the denominator and put that in the answer. Then multiply the denominator by that answer put that below the numerator and subtract to create a new polynomial. Pull down the remaining polynomials.

L4 Divide polynomials using long division. This is a one-sided practice page with 9 questions on Dividing a Polynomial by a Monomial. After dividing each polynomial students use a coloring key to color a tessellation design.

Coloring is calming and makes the work more fun. The coloring key includes 3 extra answers that wont be used.